2021-08-28 Daily-Challenge

Posted on:
Posted modified:
In: DailyChallenge

Today is Saturday, I gonna review the tasks I've done this week, and finish today's leetcode's August LeetCoding Challenge with cpp.

LeetCode Review

Longest Uncommon Subsequence II

too easy to review

Verify Preorder Serialization of a Binary Tree

too easy to review

Sum of Square Numbers

too easy to review

Complex Number Multiplication

too easy to review

Two Sum IV - Input is a BST

too easy to review

Implement Stack using Queues

too easy to review

Design Circular Queue

too easy to review

Expressive Words

too easy to review

Sum Root to Leaf Numbers

too easy to review

Two Sum II - Input array is sorted

too easy to review

August LeetCoding Challenge 28

Description

Maximum Profit in Job Scheduling

We have n jobs, where every job is scheduled to be done from startTime[i] to endTime[i], obtaining a profit of profit[i].

You're given the startTime, endTime and profit arrays, return the maximum profit you can take such that there are no two jobs in the subset with overlapping time range.

If you choose a job that ends at time X you will be able to start another job that starts at time X.

Example 1:

img

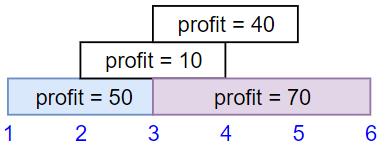
Input: startTime = [1,2,3,3], endTime = [3,4,5,6], profit = [50,10,40,70]
Output: 120
Explanation: The subset chosen is the first and fourth job. 
Time range [1-3]+[3-6] , we get profit of 120 = 50 + 70.

Example 2:

img

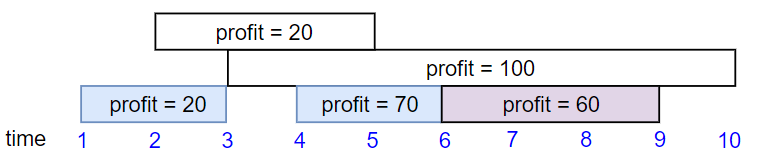
Input: startTime = [1,2,3,4,6], endTime = [3,5,10,6,9], profit = [20,20,100,70,60]
Output: 150
Explanation: The subset chosen is the first, fourth and fifth job. 
Profit obtained 150 = 20 + 70 + 60.

Example 3:

img

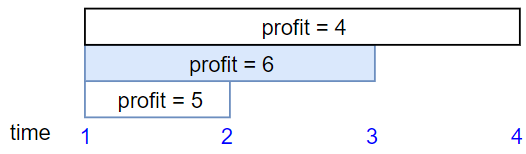
Input: startTime = [1,1,1], endTime = [2,3,4], profit = [5,6,4]
Output: 6

Constraints:

  • 1 <= startTime.length == endTime.length == profit.length <= 5 * 10^4
  • 1 <= startTime[i] < endTime[i] <= 10^9
  • 1 <= profit[i] <= 10^4

Solution

auto speedup = [](){
  cin.tie(nullptr);
  cout.tie(nullptr);
  ios::sync_with_stdio(false);
  return 0;
}();
struct Job {
  int start;
  int end;
  int profit;
  Job() {}
  Job(int start, int end, int profit): start(start), end(end), profit(profit) {}
  bool operator<(const Job& other) const { 
    return this->start < other.start;
  }
};
class Solution {
public:
  int jobScheduling(vector<int>& startTime, vector<int>& endTime, vector<int>& profit) {
    vector<Job> jobs;
    int len = startTime.size();
    for(int i = 0; i < startTime.size(); i++) {
      jobs.push_back(Job(startTime[i], endTime[i], profit[i]));
    }
    sort(jobs.begin(), jobs.end());
    vector<int> dp(len);
    for(int i = 0; i < len; ++i) {
      dp[i] = jobs[i].profit;
    }
    for(int i = 0; i < len; i++) {
      if(i) {
        dp[i] = max(dp[i], dp[i - 1] - jobs[i - 1].profit + jobs[i].profit);
      }
      int pos = lower_bound(jobs.begin() + i, jobs.end(), jobs[i].end, 
        [](const Job& j, int endTime) {
          return j.start < endTime;
        }) - jobs.begin();
      if(pos < len && dp[pos] < dp[i] + jobs[pos].profit) dp[pos] = dp[i] + jobs[pos].profit;
    }
    return *max_element(dp.begin(), dp.end());
  }
};

// Accepted
// 27/27 cases passed (60 ms)
// Your runtime beats 99.91 % of cpp submissions
// Your memory usage beats 77.26 % of cpp submissions (49.7 MB)
Algorithm