Today I have done Min Stack and leetcode's August LeetCoding Challenge with cpp.

Min Stack

Description

Design a stack that supports push, pop, top, and retrieving the minimum element in constant time.

Implement the MinStack class:

• MinStack() initializes the stack object.
• void push(val) pushes the element val onto the stack.
• void pop() removes the element on the top of the stack.
• int top() gets the top element of the stack.
• int getMin() retrieves the minimum element in the stack.

Example 1:

Input
["MinStack","push","push","push","getMin","pop","top","getMin"]
[[],[-2],[0],[-3],[],[],[],[]]

Output
[null,null,null,null,-3,null,0,-2]

Explanation
MinStack minStack = new MinStack();
minStack.push(-2);
minStack.push(0);
minStack.push(-3);
minStack.getMin(); // return -3
minStack.pop();
minStack.top();    // return 0
minStack.getMin(); // return -2

Constraints:

• -231 <= val <= 231 - 1
• Methods pop, top and getMin operations will always be called on non-empty stacks.
• At most 3 * 104 calls will be made to push, pop, top, and getMin.

Solution

auto speedup = [](){
  cin.tie(nullptr);
  cout.tie(nullptr);
  ios::sync_with_stdio(false);
  return 0;
}();
class MinStack {
  vector<int> st;
  vector<int> minSt;
public:
  /** initialize your data structure here. */
  MinStack(){}
  
  void push(int val) {
    st.push_back(val);
    if(minSt.empty() || val <= minSt.back()) {
      minSt.push_back(val);
    }
  }
  
  void pop() {
    if(st.back() == minSt.back()) {
      minSt.pop_back();
    }
    st.pop_back();
  }
  
  int top() {
    return st.back();
  }
  
  int getMin() {
    return minSt.back();
  }
};

// Accepted
// 31/31 cases passed (12 ms)
// Your runtime beats 99.73 % of cpp submissions
// Your memory usage beats 33.29 % of cpp submissions (16.5 MB)

August LeetCoding Challenge 19

Description

Maximum Product of Splitted Binary Tree

Given the root of a binary tree, split the binary tree into two subtrees by removing one edge such that the product of the sums of the subtrees is maximized.

Return the maximum product of the sums of the two subtrees. Since the answer may be too large, return it modulo 109 + 7.

Note that you need to maximize the answer before taking the mod and not after taking it.

Example 1:

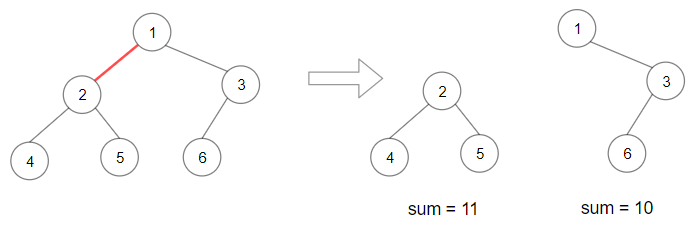
Input: root = [1,2,3,4,5,6]
Output: 110
Explanation: Remove the red edge and get 2 binary trees with sum 11 and 10. Their product is 110 (11*10)

Example 2:

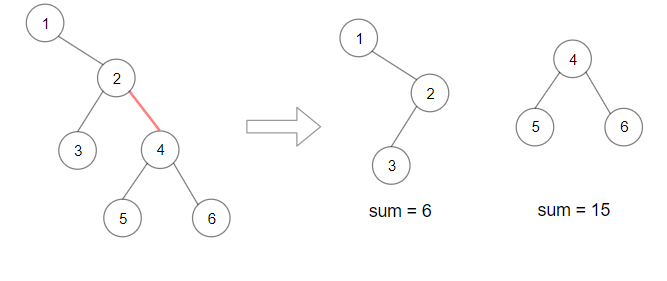
Input: root = [1,null,2,3,4,null,null,5,6]
Output: 90
Explanation: Remove the red edge and get 2 binary trees with sum 15 and 6.Their product is 90 (15*6)

Example 3:

Input: root = [2,3,9,10,7,8,6,5,4,11,1]
Output: 1025

Example 4:

Input: root = [1,1]
Output: 1

Constraints:

• The number of nodes in the tree is in the range [2, 5 * 10^4].
• 1 <= Node.val <= 10^4

Solution

auto speedup = [](){
  cin.tie(nullptr);
  cout.tie(nullptr);
  ios::sync_with_stdio(false);
  return 0;
}();
const int MOD = 1e9 + 7;
class Solution {
  int sum = 0;
  int cur = 0;
  void add(TreeNode *root) {
    if(!root) return;
    sum += root->val;
    add(root->left);
    add(root->right);
  }
  int solve(TreeNode *root) {
    if(!root) return 0;
    int left = solve(root->left);
    int right = solve(root->right);
    if(abs(left + right + root->val - sum / 2) < abs(cur - sum / 2)) {
      cur = left + right + root->val;
    }
    if (abs(left - sum / 2) < abs(cur - sum / 2)) {
      cur = left;
    }
    if (abs(right - sum / 2) < abs(cur - sum / 2)) {
      cur = right;
    }
    return left + right + root->val;
  }
public:
  int maxProduct(TreeNode* root) {
    add(root);
    solve(root);
    return 1LL * (sum - cur) * cur % MOD;
  }
};

// Accepted
// 54/54 cases passed (84 ms)
// Your runtime beats 100 % of cpp submissions
// Your memory usage beats 63.53 % of cpp submissions (77.5 MB)