Today I have done Rank Transform of an Array and leetcode's July LeetCoding Challenge with cpp.

Rank Transform of an Array

Description

Given an array of integers arr, replace each element with its rank.

The rank represents how large the element is. The rank has the following rules:

• Rank is an integer starting from 1.
• The larger the element, the larger the rank. If two elements are equal, their rank must be the same.
• Rank should be as small as possible.

Example 1:

Input: arr = [40,10,20,30]
Output: [4,1,2,3]
Explanation: 40 is the largest element. 10 is the smallest. 20 is the second smallest. 30 is the third smallest.

Example 2:

Input: arr = [100,100,100]
Output: [1,1,1]
Explanation: Same elements share the same rank.

Example 3:

Input: arr = [37,12,28,9,100,56,80,5,12]
Output: [5,3,4,2,8,6,7,1,3]

Constraints:

• 0 <= arr.length <= 10^5
• -10^9 <= arr[i] <= 10^9

Solution

bad data, counting sort can be 44ms and beat 100%, but it's a fake solution.

class Solution {
public:
  vector<int> arrayRankTransform(vector<int>& arr) {
    vector<int> order(arr);
    sort(order.begin(), order.end());
    order.resize(unique(order.begin(), order.end()) - order.begin());
    unordered_map<int, int> rk;
    int count = 0;
    for(auto i : order) rk[i] = ++count;
    for(auto &i : arr) i = rk[i];
    return arr;
  }
};

// Accepted
// 37/37 cases passed (84 ms)
// Your runtime beats 89.57 % of cpp submissions
// Your memory usage beats 72.51 % of cpp submissions (39.1 MB)

July LeetCoding Challenge 19

Description

Lowest Common Ancestor of a Binary Search Tree

Given a binary search tree (BST), find the lowest common ancestor (LCA) of two given nodes in the BST.

According to the definition of LCA on Wikipedia: “The lowest common ancestor is defined between two nodes p and q as the lowest node in T that has both p and q as descendants (where we allow a node to be a descendant of itself).”

Example 1:

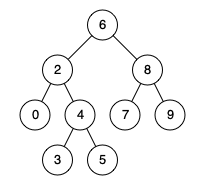
Input: root = [6,2,8,0,4,7,9,null,null,3,5], p = 2, q = 8
Output: 6
Explanation: The LCA of nodes 2 and 8 is 6.

Example 2:

Input: root = [6,2,8,0,4,7,9,null,null,3,5], p = 2, q = 4
Output: 2
Explanation: The LCA of nodes 2 and 4 is 2, since a node can be a descendant of itself according to the LCA definition.

Example 3:

Input: root = [2,1], p = 2, q = 1
Output: 2

Constraints:

• The number of nodes in the tree is in the range [2, 10^5].
• -10^9 <= Node.val <= 10^9
• All Node.val are unique.
• p != q
• p and q will exist in the BST.

Solution

class Solution {
public:
  TreeNode* lowestCommonAncestor(TreeNode* root, TreeNode* p, TreeNode* q) {
    if(!root || root == p || root == q) return root;
    TreeNode *left = lowestCommonAncestor(root->left, p, q);
    TreeNode *right = lowestCommonAncestor(root->right, p, q);
    if(left && right) return root;
    if(left) return left;
    if(right) return right;
    return nullptr;
  }
};

// Accepted
// 27/27 cases passed (28 ms)
// Your runtime beats 79.85 % of cpp submissions
// Your memory usage beats 9.47 % of cpp submissions (23.3 MB)

ah ha, it's a BST

auto speedup = [](){
  cin.tie(nullptr);
  cout.tie(nullptr);
  ios::sync_with_stdio(false);
  return 0;
}();
class Solution {
public:
  TreeNode* lowestCommonAncestor(TreeNode* root, TreeNode* p, TreeNode* q) {
    if(!root) return root;
    if(root->val < p->val && root->val < q->val) return lowestCommonAncestor(root->right, p, q);
    else if(root->val > p->val && root->val > q->val) return lowestCommonAncestor(root->left, p, q);
    return root;
  }
};

// Accepted
// 27/27 cases passed (20 ms)
// Your runtime beats 99.02 % of cpp submissions
// Your memory usage beats 57.6 % of cpp submissions (23.3 MB)