Today I have done Guess the Word and leetcode's June LeetCoding Challenge with cpp.

Guess the Word

Description

This is an interactive problem.

You are given an array of unique strings wordlist where wordlist[i] is 6 letters long, and one word in this list is chosen as secret.

You may call Master.guess(word) to guess a word. The guessed word should have type string and must be from the original list with 6 lowercase letters.

This function returns an integer type, representing the number of exact matches (value and position) of your guess to the secret word. Also, if your guess is not in the given wordlist, it will return -1 instead.

For each test case, you have exactly 10 guesses to guess the word. At the end of any number of calls, if you have made 10 or fewer calls to Master.guess and at least one of these guesses was secret, then you pass the test case.

Example 1:

Input: secret = "acckzz", wordlist = ["acckzz","ccbazz","eiowzz","abcczz"], numguesses = 10
Output: You guessed the secret word correctly.
Explanation:
master.guess("aaaaaa") returns -1, because "aaaaaa" is not in wordlist.
master.guess("acckzz") returns 6, because "acckzz" is secret and has all 6 matches.
master.guess("ccbazz") returns 3, because "ccbazz" has 3 matches.
master.guess("eiowzz") returns 2, because "eiowzz" has 2 matches.
master.guess("abcczz") returns 4, because "abcczz" has 4 matches.
We made 5 calls to master.guess and one of them was the secret, so we pass the test case.

Example 2:

Input: secret = "hamada", wordlist = ["hamada","khaled"], numguesses = 10
Output: You guessed the secret word correctly.

Constraints:

• 1 <= wordlist.length <= 100
• wordlist[i].length == 6
• wordlist[i] consist of lowercase English letters.
• All the strings of wordlist are unique.
• secret exists in wordlist.
• numguesses == 10

Solution

const int N = 6;
inline int match(const string &a, const string &b) {
  int result = 0;
  for(int i = 0; i < 6; ++i) {
    result += a[i] == b[i];
  }
  return result;
}
class Solution {
  vector<vector<int>> adj;
  int len;
  void init(vector<string> & wordlist) {
    len = wordlist.size();
    adj.resize(len, vector<int>(len));
    for(int i = 0; i < len; ++i) {
      for(int j = i + 1; j < len; ++j) {
        adj[i][j] = adj[j][i] = match(wordlist[i], wordlist[j]);
      }
    }
    for(int i = 0; i < len; ++i) {
      for(int j = 0; j < len; ++j) {
        if(i == j) continue;
        adj[i][i] += adj[i][j];
      }
    }
  }

  int getNextGuess() {
    int idx = -1;
    int maxAdj = -1;
    for(int i = 0; i < len; ++i) {
      if(adj[i][i] > maxAdj) {
        idx = i;
        maxAdj = adj[i][i];
      }
    }
    return idx;
  }

  void pruneGuess(int idx, int guess) {
    vector<int> toRemove{idx};
    if(!guess) {
      for(int i = 0; i < len; ++i) {
        if(adj[i][idx] > 0) toRemove.push_back(i);
      }
    } else {
      for(int i = 0; i < len; ++i) {
        if(adj[i][idx] < guess) toRemove.push_back(i);
      }
    }
    for(auto i : toRemove) {
      adj[i][i] = -1;
    }
  }
public:
  void findSecretWord(vector<string>& wordlist, Master& master) {
    if(wordlist.size() < 11) {
      for(auto &s : wordlist) master.guess(s);
      return;
    }
    init(wordlist);
    for(int i = 0; i < 10; ++i) {
      int g = getNextGuess();
      int result = master.guess(wordlist[g]);
      // cout << g << ' ' << result << ' ' << wordlist[g] << endl;
      if(result == 6) break;
      pruneGuess(g, result);
    }
  }
};

June LeetCoding Challenge 24

Description

Out of Boundary Paths

There is an m x n grid with a ball. The ball is initially at the position [startRow, startColumn]. You are allowed to move the ball to one of the four adjacent four cells in the grid (possibly out of the grid crossing the grid boundary). You can apply at most maxMove moves to the ball.

Given the five integers m, n, maxMove, startRow, startColumn, return the number of paths to move the ball out of the grid boundary. Since the answer can be very large, return it modulo 109 + 7.

Example 1:

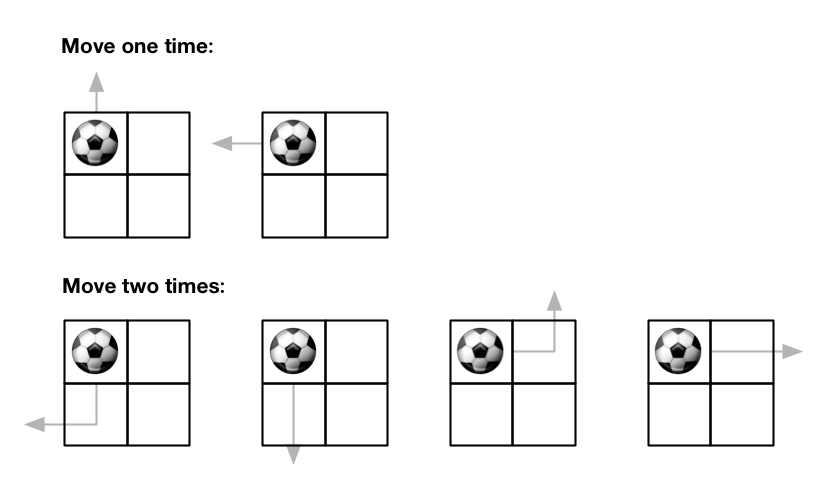
Input: m = 2, n = 2, maxMove = 2, startRow = 0, startColumn = 0
Output: 6

Example 2:

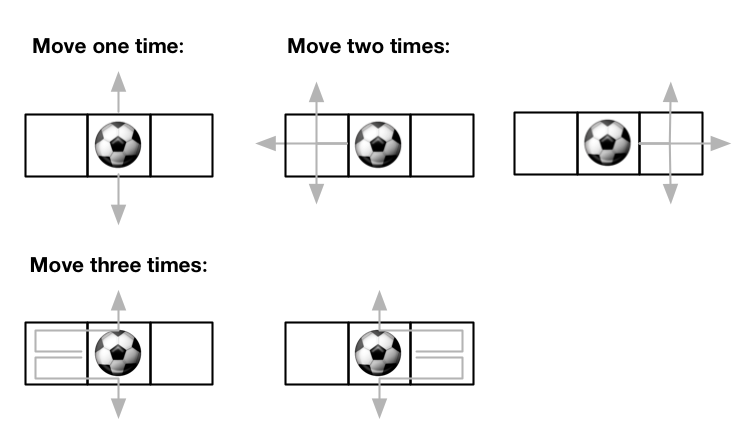
Input: m = 1, n = 3, maxMove = 3, startRow = 0, startColumn = 1
Output: 12

Constraints:

• 1 <= m, n <= 50
• 0 <= maxMove <= 50
• 0 <= startRow <= m
• 0 <= startColumn <= n

Solution

const int SZ = 50;
const int MOD = 1e9 + 7;
int path[2][SZ][SZ];
const int moves[4][2] = {{0, 1}, {1, 0}, {0, -1}, {-1, 0}};
class Solution {
public:
  int findPaths(int m, int n, int maxMove, int startRow, int startColumn) {
    memset(path[0], 0, sizeof(path[0]));
    path[0][startRow][startColumn] = 1;
    int result = 0;
    for(int cnt = 0; cnt < maxMove; cnt++) {
      int parity = cnt & 1;
      memset(path[!parity], 0, sizeof(path[0]));
      for(int i = 0; i < m; ++i) {
        for(int j = 0; j < n; ++j) {
          // cout << path[parity][i][j] << ' ' ;
          for(int c = 0; c < 4; ++c) {
            int newRow = i + moves[c][0];
            int newCol = j + moves[c][1];
            if(newRow < 0 || newCol < 0 || newRow >= m || newCol >= n) continue;
            path[!parity][newRow][newCol] += path[parity][i][j];
            path[!parity][newRow][newCol] %= MOD;
          }
        }
        // cout << endl;
      }
      // cout << endl;
      for(int i = 0; i < m; ++i) {
        result += path[parity][i][0];
        result %= MOD;
        result += path[parity][i][n - 1];
        result %= MOD;
      }
      for(int i = 0; i < n; ++i) {
        result += path[parity][0][i];
        result %= MOD;
        result += path[parity][m - 1][i];
        result %= MOD;
      }
    }
    return result;
  }
};