Today is Saturday, I gonna review the tasks I've done this week, and finish today's leetcode's May LeetCoding Challenge with cpp.

LeetCode Review

To Lower Case

too easy to review

Evaluate Reverse Polish Notation

too easy to review

Partitioning Into Minimum Number Of Deci-Binary Numbers

too easy to review

Maximum Product of Word Lengths

too easy to review

Maximum Erasure Value

too easy to review

Count Negative Numbers in a Sorted Matrix

too easy to review

Length of Longest Fibonacci Subsequence

too easy to review

Dice Roll Simulation

come up with DP formula by myself, no need to review

Create Maximum Number

still remember way of merge, no need to review

Minimum Difficulty of a Job Schedule

class Solution {
public:
  int minDifficulty(vector<int>& jd, int d) {
    int len = jd.size();
    if(d > len) return -1;
    if(len == d) return accumulate(jd.begin(), jd.end(), 0);
    if(d == 1) return *max_element(jd.begin(), jd.end());
    int dp[2][len];
    dp[0][0] = jd[0];
    for(int i = 1; i < len; ++i) dp[0][i] = max(jd[i], dp[0][i - 1]);
    vector<int> ms;
    for(int i = 1; i < d; ++i) {
      int parity = i & 1;
      ms.clear();
      for(int j = i; j < len; ++j) {
        dp[parity][j] = dp[!parity][j - 1] + jd[j];
        while(ms.size() && jd[ms.back()] < jd[j]) {
          int k = ms.back();
          ms.pop_back();
          dp[parity][j] = min(dp[parity][j], dp[parity][k] - jd[k] + jd[j]);
        }
        if(ms.size()) {
          dp[parity][j] = min(dp[parity][j], dp[parity][ms.back()]);
        }
        ms.push_back(j);
      }
    }
    return dp[!(d & 1)][len - 1];
  }
};

May LeetCoding Challenge 29

Description

N-Queens II

The n-queens puzzle is the problem of placing n queens on an n x n chessboard such that no two queens attack each other.

Given an integer n, return the number of distinct solutions to the n-queens puzzle.

Example 1:

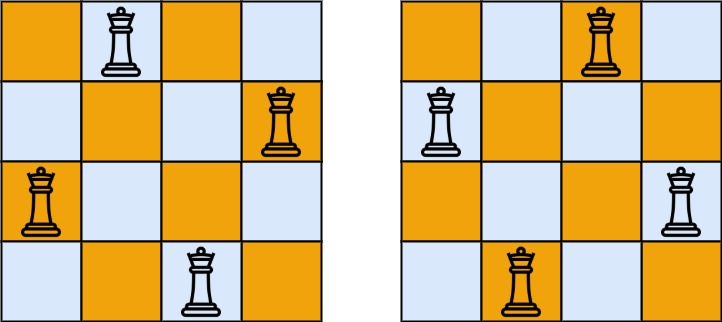
Input: n = 4
Output: 2
Explanation: There are two distinct solutions to the 4-queens puzzle as shown.

Example 2:

Input: n = 1
Output: 1

Constraints:

• 1 <= n <= 9

Solution

int rows[9];
bool check(int row, int col) {
  for(int i = 0; i < row; ++i) {
    if(abs(i - row) == abs(rows[i] - col) || col == rows[i]) return false;
  }
  return true;
}
class Solution {
  int answer = 0;

  void solve(int cur, int n) {
    if(cur == n) {
      answer += 1;
      return;
    }
    for(int i = 0; i < n; ++i) {
      if(check(cur, i)) {
        rows[cur] = i;
        solve(cur + 1, n);
      }
    }
  }
public:
  int totalNQueens(int n) {
    solve(0, n);
    return answer;
  }
};