Today I have done Count Unique Characters of All Substrings of a Given String and leetcode's April LeetCoding Challenge with cpp.

Count Unique Characters of All Substrings of a Given String

Description

Let's define a function countUniqueChars(s) that returns the number of unique characters on s, for example if s = "LEETCODE" then "L", "T","C","O","D" are the unique characters since they appear only once in s, therefore countUniqueChars(s) = 5.

On this problem given a string s we need to return the sum of countUniqueChars(t) where t is a substring of s. Notice that some substrings can be repeated so on this case you have to count the repeated ones too.

Since the answer can be very large, return the answer modulo 10 ^ 9 + 7.

Example 1:

Input: s = "ABC"
Output: 10
Explanation: All possible substrings are: "A","B","C","AB","BC" and "ABC".
Evey substring is composed with only unique letters.
Sum of lengths of all substring is 1 + 1 + 1 + 2 + 2 + 3 = 10

Example 2:

Input: s = "ABA"
Output: 8
Explanation: The same as example 1, except countUniqueChars("ABA") = 1.

Example 3:

Input: s = "LEETCODE"
Output: 92

Constraints:

• 0 <= s.length <= 10^4
• s contain upper-case English letters only.

Solution

I've drawn a picture to illustrate my solution

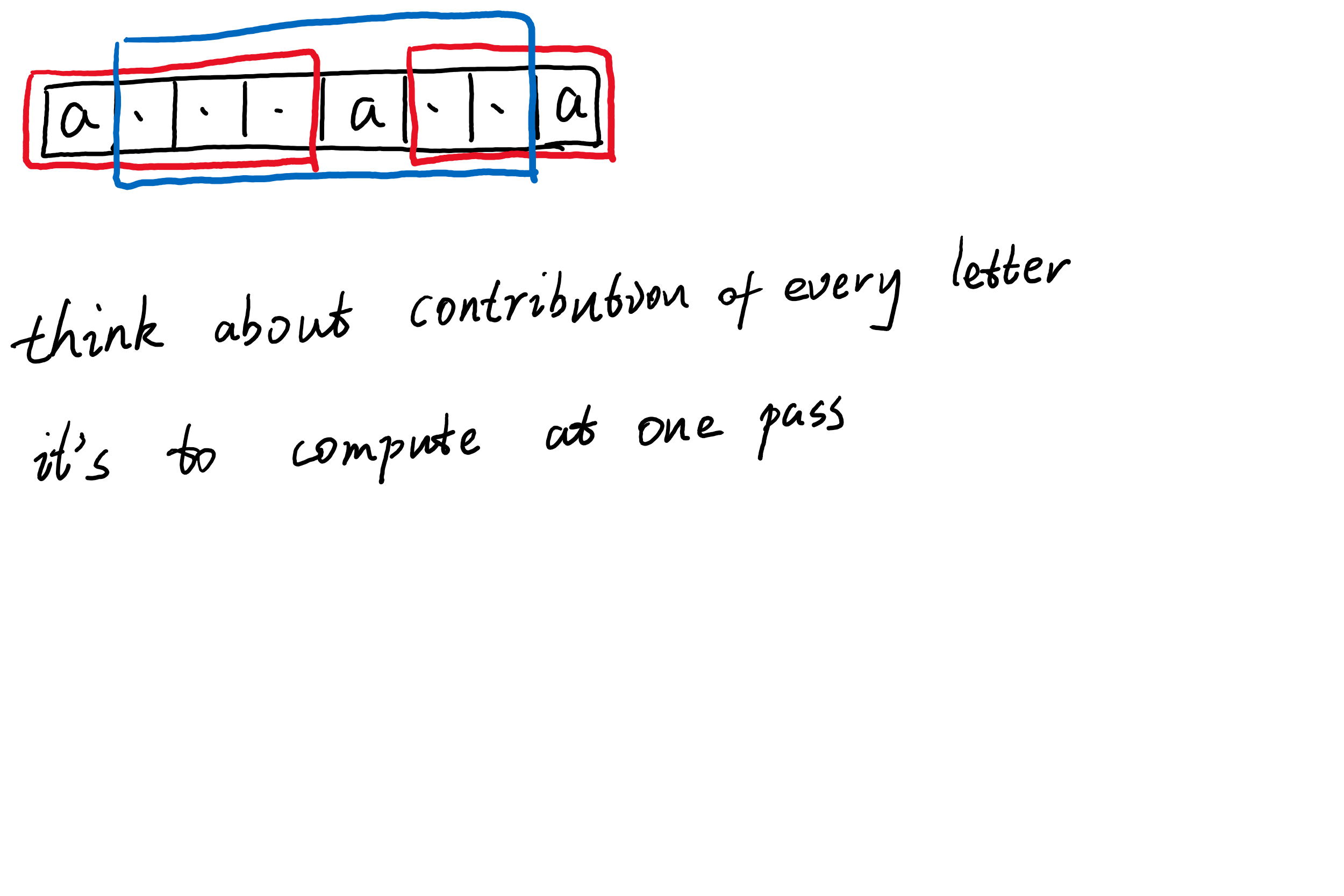
const int MOD = 1e9 + 7;

class Solution {
public:
  int uniqueLetterString(string s) {
    int len = s.length();
    int answer = 0;
    for(int i = 0; i < 26; ++i) {
      int last2 = -1;
      int last1 = -1;
      int pos = 0;
      for(; pos < len; ++pos) {
        if(s[pos] == 'A' + i) {
          answer += (last1 - last2) * (pos - last1);
          answer %= MOD;
          last2 = last1;
          last1 = pos;
        }
      }
      if(last1 != -1) {
        answer += (len - last1) * (last1 - last2);
        answer %= MOD;
      }
    }
    
    return answer;
  }
};

April LeetCoding challenge 2

Description

Ones and Zeroes

You are given an array of binary strings strs and two integers m and n.

Return the size of the largest subset of strs such that there are at most m 0's and n 1's in the subset.

A set x is a subset of a set y if all elements of x are also elements of y.

Example 1:

Input: strs = ["10","0001","111001","1","0"], m = 5, n = 3
Output: 4
Explanation: The largest subset with at most 5 0's and 3 1's is {"10", "0001", "1", "0"}, so the answer is 4.
Other valid but smaller subsets include {"0001", "1"} and {"10", "1", "0"}.
{"111001"} is an invalid subset because it contains 4 1's, greater than the maximum of 3.

Example 2:

Input: strs = ["10","0","1"], m = 1, n = 1
Output: 2
Explanation: The largest subset is {"0", "1"}, so the answer is 2.

Constraints:

• 1 <= strs.length <= 600
• 1 <= strs[i].length <= 100
• strs[i] consists only of digits '0' and '1'.
• 1 <= m, n <= 100

Solution

I come up with a solution with time complexity of $O(Accept)$, and I didn't expect it would be great solution, BUT...

class Solution {
public:
  int findMaxForm(vector<string>& strs, int m, int n) {
    int len = strs.size();
    int count[len][2];
    for(int i = 0; i < len; ++i) {
      count[i][0] = 0;
      count[i][1] = 0;
      for(auto c : strs[i]) count[i][c == '1'] += 1;
    }
    int dp[m + 1][n + 1];
    for(int i = 0; i <= m; ++i) {
      for(int j = 0; j <= n; ++j) dp[i][j] = -1;
    }
    int answer = 0;
    dp[0][0] = 0;
    for(int i = 0; i < len; ++i) {
      for(int zero = m; zero >= count[i][0]; --zero) {
        for(int one = n; one >= count[i][1]; --one) {
          if(dp[zero - count[i][0]][one - count[i][1]] == -1) continue;
          dp[zero][one] = max(dp[zero - count[i][0]][one - count[i][1]] + 1, dp[zero][one]);
          answer = max(answer, dp[zero][one]);
        }
      }
    }
    
    return answer;
  }
};

// Runtime: 44 ms, faster than 97.67% of C++ online submissions for Ones and Zeroes.
// Memory Usage: 8.6 MB, less than 99.46% of C++ online submissions for Ones and Zeroes.