Today I have done Distinct Subsequences and Distinct Subsequences II on leetcode and leetcode's December LeetCoding Challenge with cpp.

Distinct Subsequences

Description

Given two strings s and t, return the number of distinct subsequences of s which equals t.

A string's subsequence is a new string formed from the original string by deleting some (can be none) of the characters without disturbing the relative positions of the remaining characters. (i.e., "ACE" is a subsequence of "ABCDE" while "AEC" is not).

It's guaranteed the answer fits on a 32-bit signed integer.

Example 1:

Input: s = "rabbbit", t = "rabbit"
Output: 3
Explanation:
As shown below, there are 3 ways you can generate "rabbit" from S.
rabbbit
rabbbit
rabbbit

Example 2:

Input: s = "babgbag", t = "bag"
Output: 5
Explanation:
As shown below, there are 5 ways you can generate "bag" from S.
babgbag
babgbag
babgbag
babgbag
babgbag

Constraints:

• 0 <= s.length, t.length <= 1000
• s and t consist of English letters.

Solution

LCS DP, but I forgot it... fuck

class Solution {
public:
  int numDistinct(string s, string t) {
    int slen = s.length();
    int tlen = t.length();
    if(slen <= tlen) return s == t;
    vector<vector<long long>> dp(slen+1, vector<long long>(tlen+1));
    for(int i = 0; i <= slen; ++i) {
      dp[i][0] = 1;
    }
    for(int i = 1; i <= slen; ++i) {
      for(int j = max(1, tlen+i-slen-1); j <= min(slen-tlen+i, tlen); ++j) {
        dp[i][j] = dp[i-1][j];
        if(s[i-1] == t[j-1]) dp[i][j] += dp[i-1][j-1];
      }
    }
    return dp.back().back();
  }
};

Distinct Subsequences II

Description

Given a string S, count the number of distinct, non-empty subsequences of S .

Since the result may be large, return the answer modulo 10^9 + 7.

Example 1:

Input: "abc"
Output: 7
Explanation: The 7 distinct subsequences are "a", "b", "c", "ab", "ac", "bc", and "abc".

Example 2:

Input: "aba"
Output: 6
Explanation: The 6 distinct subsequences are "a", "b", "ab", "ba", "aa" and "aba".

Example 3:

Input: "aaa"
Output: 3
Explanation: The 3 distinct subsequences are "a", "aa" and "aaa".

Note:

1. S contains only lowercase letters.
2. 1 <= S.length <= 2000

Solution

little thing similar to previous problem, check solution for more information.

class Solution {
public:
    int distinctSubseqII(string S) {
        const int MOD = 1000000007;
        int len = S.length();
        vector<int> dp(len+1);
        dp[0] = 1;
        vector<int> last(26, -1);
        for(int i = 1; i <= len; ++i) {
            dp[i] = dp[i-1] * 2 % MOD;
            int c = S[i-1] - 'a';
            if(last[c] != -1) {
                dp[i] -= dp[last[c]];
            }
            dp[i] = (dp[i] + MOD) % MOD;
            last[c] = i-1;
        }
        dp[len] = (dp[len] - 1 + MOD) % MOD;
        return dp.back();
    }
};

December LeetCoding Challenge 9

Description

Binary Search Tree Iterator

Implement the BSTIterator class that represents an iterator over the in-order traversal of a binary search tree (BST):

• BSTIterator(TreeNode root) Initializes an object of the BSTIterator class. The root of the BST is given as part of the constructor. The pointer should be initialized to a non-existent number smaller than any element in the BST.
• boolean hasNext() Returns true if there exists a number in the traversal to the right of the pointer, otherwise returns false.
• int next() Moves the pointer to the right, then returns the number at the pointer.

Notice that by initializing the pointer to a non-existent smallest number, the first call to next() will return the smallest element in the BST.

You may assume that next() calls will always be valid. That is, there will be at least a next number in the in-order traversal when next() is called.

Example 1:

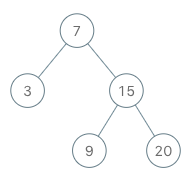
Input
["BSTIterator", "next", "next", "hasNext", "next", "hasNext", "next", "hasNext", "next", "hasNext"]
[[[7, 3, 15, null, null, 9, 20]], [], [], [], [], [], [], [], [], []]
Output
[null, 3, 7, true, 9, true, 15, true, 20, false]

Explanation
BSTIterator bSTIterator = new BSTIterator([7, 3, 15, null, null, 9, 20]);
bSTIterator.next();    // return 3
bSTIterator.next();    // return 7
bSTIterator.hasNext(); // return True
bSTIterator.next();    // return 9
bSTIterator.hasNext(); // return True
bSTIterator.next();    // return 15
bSTIterator.hasNext(); // return True
bSTIterator.next();    // return 20
bSTIterator.hasNext(); // return False

Constraints:

• The number of nodes in the tree is in the range [1, 105].
• 0 <= Node.val <= 106
• At most 105 calls will be made to hasNext, and next.

Follow up:

• Could you implement next() and hasNext() to run in average O(1) time and use O(h) memory, where h is the height of the tree?

Solution

nothing to say

class BSTIterator {
    vector<TreeNode*> previous;
    TreeNode* cur;
public:
    BSTIterator(TreeNode* root) {
        while(root && root->left) {
            previous.push_back(root);
            root = root->left;
        }
        cur = root;
    }
    
    int next() {
        int result = cur->val;
        if(cur->right) {
            TreeNode *root = cur->right;
            while(root && root->left) {
                previous.push_back(root);
                root = root->left;
            }
            cur = root;
        } else {
            if(previous.size()) {
                cur = previous.back();
                previous.pop_back();
            } else {
                cur = nullptr;
            }
        }
        return result;
    }
    
    bool hasNext() {
        return cur;
    }
};